What Number Is Your Henge Should Stone Way?

{\displaystyle {\boldsymbol {T}}_{o}(p_{i,j})=p_{i,j}+t_{o}}

Listen to George Noory this evening this lovely June of a year that declares that Pluto may be the ear to the spacial belt that wraps this galaxy at a base.  Wormhole on that and announce!  The micro-fear of our Earth has dismissed a Comet for the Planet and charged a Star for it's fee.

Touching this back as the spine in the vertebrae the fluid joint would gain.  The snapping effect to moth not butterfly would round an Orion to touch cosmos and knee the joint on a balance that mirror reflect.  To grain of sand, Coast to Coast, an Anchor with the call-in taking his answer off-air.  Ground zero would be Mankind as Man Himself.

Change the detail and envelope another card to Post the office and address a tar.(?).  Would that not be the Valley of mountainous land, the Forest in the clam asking Muscles do you lost.  Basic to slow as George Noory spoke, 49 Years:  Since the answer to The Moon.  Oh for the voice a vocal deep bland, echo at groves and beacons to Crows.

KGO 810 Radio AM San Francisco the number of say is George Noory today!!  The month is of June, the season so Summer, does July just be claim as the 4th be it's bane?  What of this be Grand Canyon to sand should the 'What if' been dropped from Friday?  Then Thursday would wheel to Saturday?  Nigh these hours to Minutemen harness as a bridle for dinner and a level for Hearst.

Newspaper wrappers are called rubber bands.  The front door deliver to now cars in the hand.  Drive Shafts or buses the seat is of BART now that cell phones and texts are in competition and Band.

Sing is a note yet the verse is of words, is the type written scale the Bass or the Fan!!  The Clef.

Angular velocity - Wikipedia

https://en.wikipedia.org/wiki/Angular_velocity

In physics, the angular velocity of a particle is the rate at which it rotates around a chosen ... vector r from the origin O to the particle P, with its polar coordinates ( r , ϕ ) {\displaystyle (r,\phi )} {\displaystyle (r,\phi )} . (All variables are functions of time t.) ...

Transposition (music) - Wikipedia

https://en.wikipedia.org/wiki/Transposition_(music)

In music transposition refers to the process, or operation, of moving a collection of notes ... The transposition of a set A by n semitones is designated by Tn(A), representing .... {T}}_{o}(p_{i,j})=p_{i,j}+t_{o}} {\displaystyle {\boldsymbol {T}}_{o}(p_ ... to to (or o, according to Schuijer); pi,j is the pitch of the ith tone in P belong to the ...

Langevin equation - Wikipedia

https://en.wikipedia.org/wiki/Langevin_equation

In physics, Langevin equation (named after Paul Langevin) is a stochastic differential equation .... However, the Langevin equation is used to describe the motion of a ... isn't defined in this limit. ..... Other universality classes (the nomenclature is "model A",..., "model J") contain a ... {\displaystyle \lambda {\frac {d\left\langle f(.

Missing: o

Transposition (music)

From Wikipedia, the free encyclopedia

  (Redirected from Sight transposition)

Jump to navigation Jump to search

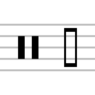
Transposition example from Koch^[1] 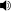 Play top (help·info)  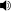Play bottom (help·info). The melody on the first line is in the key of D, while the melody on the second line is identical except that it is major third lower, in the key of B♭.

In music transposition refers to the process, or operation, of moving a collection of notes (pitches or pitch classes) up or down in pitch by a constant interval.

The shifting of a melody, a harmonic progression or an entire musical piece to another key, while maintaining the same tone structure, i.e. the same succession of whole tones and semitones and remaining melodic intervals.

— Musikalisches Lexicon, 879 (1865), Heinrich Christoph Koch (trans. Schuijer)^[1]

For example, one might transpose an entire piece of music into another key. Similarly, one might transpose a tone row or an unordered collection of pitches such as a chord so that it begins on another pitch.
The transposition of a set A by n semitones is designated by T_n(A), representing the addition (mod 12) of an integer n to each of the pitch class integers of the set A.^[1] Thus the set (A) consisting of 0–1–2 transposed by 5 semitones is 5–6–7 (T₅(A)) since 0 + 5 = 5, 1 + 5 = 6, and 2 + 5 = 7.

Contents

• 1 Four kinds of transposition
  • 1.1 Chromatic and scalar (diatonic) transposition
    • 1.1.1 Chromatic
    • 1.1.2 Scalar
  • 1.2 Pitch and pitch class
• 2 Sight transposition
  • 2.1 Interval
  • 2.2 Clef
  • 2.3 Numbers
• 3 Transpositional equivalence
• 4 Twelve-tone transposition
• 5 Fuzzy transposition
• 6 See also
• 7 Sources
• 8 External links

Four kinds of transposition

Chromatic and scalar (diatonic) transposition

There are two different kinds of transposition, depending on whether one is measuring intervals according to the chromatic scale or some other scale.

Chromatic

In chromatic transposition one shifts every pitch in a collection of notes by a fixed number of semitones. For instance, if one transposes the pitches C₄–E₄–G₄ upwards by four semitones, one obtains the pitches E₄–G♯₄–B₄.

Scalar

In scalar transposition one shifts every pitch in a collection by a fixed number of scale steps relative to some scale. For example, if one transposes the pitches C₄–E₄–G₄ up by two steps relative to the familiar C major scale, one obtains the pitches E₄–G₄–B₄. If one transposes the same pitches up by two steps relative to the F major scale, one obtains instead E₄–G₄–B♭₄. Scalar transposition is sometimes called diatonic transposition, but this term can be misleading, as it suggests transposition with respect to a diatonic scale. However, scalar transposition can occur with respect to any type of scale, not just the diatonic.

Pitch and pitch class

There are two further kinds of transposition, by pitch interval or by pitch interval class, applied to pitches or pitch classes, respectively. Transposition may be applied to pitches or to pitch classes.^[1] For example, the pitch A₄, or 9, transposed by a major third, or the pitch interval 4:

while that pitch class, 9, transposed by a major fourth, or the pitch class interval 4:

.

Sight transposition

Excerpt of the trumpet part of Symphony No. 9 of Antonín Dvořák, where sight transposition is required.

Although transpositions are usually written out, musicians are occasionally asked to transpose music "at sight", that is, to read the music in one key while playing in another. Musicians who play transposing instruments sometimes have to do this (for example when encountering an unusual transposition, such as clarinet in C), as well as singers' accompanists, since singers sometimes request a different key than the one printed in the music to better fit their vocal range (although many, but not all, songs are printed in editions for high, medium, and low voice).
There are three basic techniques for teaching sight transposition: interval, clef, and numbers.

Interval

First one determines the interval between the written key and the target key. Then one imagines the notes up (or down) by the corresponding interval. A performer using this method may calculate each note individually, or group notes together (e.g. "a descending chromatic passage starting on F" might become a "descending chromatic passage starting on A" in the target key).

Clef

Clef transposition is routinely taught (among other places) in Belgium and France. One imagines a different clef and a different key signature than the ones printed. The change of clef is used so that the lines and spaces correspond to different notes than the lines and spaces of the original score. Seven clefs are used for this: treble (2nd line G-clef), bass (4th line F-clef), baritone (3rd line F-clef or 5th line C-clef, although in France and Belgium sight-reading exercises for this clef, as a preparation for clef transposition practice, are always printed with the 3rd line F-clef), and C-clefs on the four lowest lines; these allow any given staff position to correspond to each of the seven note names A through G. The signature is then adjusted for the actual accidental (natural, sharp or flat) one wants on that note. The octave may also have to be adjusted (this sort of practice ignores the conventional octave implication of the clefs), but this is a trivial matter for most musicians.

Numbers

Transposing by numbers means, one determines the scale degree of the written note (e.g. first, fourth, fifth, etc.) in the given key. The performer then plays the corresponding scale degree of the target chord.

Transpositional equivalence

Two musical objects are transpositionally equivalent if one can be transformed into another by transposition. It is similar to enharmonic equivalence and octave equivalence. In many musical contexts, transpositionally equivalent chords are thought to be similar. Transpositional equivalence is a feature of musical set theory. The terms transposition and transposition equivalence allow the concept to be discussed as both an operation and relation, an activity and a state of being. Compare with modulation and related key.
Using integer notation and modulo 12, to transpose a pitch x by n semitones:

or

For pitch class transposition by a pitch class interval:

^[2]

Twelve-tone transposition

Milton Babbitt defined the "transformation" of transposition within the twelve-tone technique as follows: By applying the transposition operator (T) to a [twelve-tone] set we will mean that every p of the set P is mapped homomorphically (with regard to order) into a T(p) of the set T(P) according to the following operation:

where t_o is any integer 0–11 inclusive, where, of course, the t_o remains fixed for a given transposition. The + sign indicates ordinary transposition. Here T_o is the transposition corresponding to t_o (or o, according to Schuijer); p_i,j is the pitch of the ith tone in P belong to the pitch class (set number) j.

^[3]

Allen Forte defines transposition so as to apply to unordered sets of other than twelve pitches:

the addition mod 12 of any integer k in S to every integer p of P.

thus giving, "12 transposed forms of P".^[4]

Fuzzy transposition

Straus created the concept of fuzzy transposition, and fuzzy inversion, to express transposition as a voice-leading event, "the 'sending' of each element of a given PC [pitch-class] set to its T_n-correspondent...[enabling] him to relate PC sets of two adjacent chords in terms of a transposition, even when not all of the 'voices' participated fully in the transpositional move.".^[5] A transformation within voice-leading space rather than pitch-class space as in pitch class transposition.

See also

• Modulation (music)
• Pitch shift
• Transposing instrument

Clef

From Wikipedia, the free encyclopedia

Jump to navigation Jump to search

For other uses, see Clef (disambiguation).

This article has multiple issues. Please help improve it or discuss these issues on the talk page. (Learn how and when to remove these template messages) This article needs additional citations for verification. (April 2014) This article includes a list of references, but its sources remain unclear because it has insufficient inline citations. (April 2014)

Treble and bass clefs shown with names of the notes.

A clef (from French: clef "key") is a musical symbol used to indicate the pitch of written notes.^[1] Placed on one of the lines at the beginning of the stave, it indicates the name and pitch of the notes on that line. This line serves as a reference point by which the names of the notes on any other line or space of the stave may be determined. Only one clef that references a note in a space rather than on a line has ever been used.
There are three types of clef used in modern music notation: F, C, and G. Each type of clef assigns a different reference note to the line (and in rare cases, the space) on which it is placed. (G and F clefs are placed as treble and bass clefs, respectively, in the vast majority of modern music.)

Clef	Name	Note	Line
	G-clef	G4	passes through the curl of the clef
	C-clef	C4 (Middle C)	passes through the centre of the clef
	F-clef	F3	passes between the two dots of the clef

Once one of these clefs has been placed on one of the lines of the stave, the other lines and spaces can be read in relation to it.
The use of three different clefs makes it possible to write music for all instruments and voices, even though they may have very different tessituras. This would be difficult to do with only one clef, since the modern stave has only five lines, and the number of pitches that can be represented on the stave, even with ledger lines, is not nearly equal to the number of notes the orchestra can produce. The use of different clefs for various instruments and voices allows each part to be written comfortably on the stave with a minimum of ledger lines. To this end, the G-clef is used for high parts, the C-clef for middle parts, and the F-clef for low parts—with the notable exception of transposing parts, which are written at a pitch different from their sound, often even in a different octave.

Contents

• 1 Placement on the stave
• 2 Individual clefs
  • 2.1 G-clefs
    • 2.1.1 Treble clef
    • 2.1.2 French violin clef^†
  • 2.2 F-clefs
    • 2.2.1 Bass clef
    • 2.2.2 Baritone clef^†
    • 2.2.3 Sub-bass clef^†
  • 2.3 C-clefs
    • 2.3.1 Alto clef
    • 2.3.2 Tenor clef
    • 2.3.3 Baritone clef^†
    • 2.3.4 Mezzo-soprano clef^†
    • 2.3.5 Soprano clef^†
• 3 Other clefs
  • 3.1 Octave clefs
  • 3.2 Neutral clef
  • 3.3 Tablature
• 4 History
• 5 Further uses
• 6 See also
• 7 Notes
• 8 References
• 9 Further reading
• 10 External links

Placement on the stave

To facilitate writing for different tessituras, any of the clefs may theoretically be placed on any of the lines of the stave. The further down on the stave a clef is placed, the higher the tessitura it is for; conversely, the higher up the clef, the lower the tessitura.
Since there are five lines on the stave, and three clefs, it might seem that there would be fifteen possible clefs. Six of these, however, are redundant clefs (for example, a G-clef on the third line would be the same as a C-clef on the first line). That leaves nine possible distinct clefs, all of which have been used historically: the G-clef on the two bottom lines, the F-clef on the three top lines, and the C-clef on any line of the stave except the topmost, earning the name of "movable C-clef". (The C-clef on the topmost line is redundant because it is equivalent to the F-clef on the third line; both options have been used.)
Each of these clefs has a different name based on the tessitura for which it is best suited.

In modern music, only four clefs are used regularly: the treble clef, the bass clef, the alto clef, and the tenor clef. Of these, the treble and bass clefs are by far the most common. The tenor clef is used for the upper register of several instruments that usually use bass clef (such as cello or trombone), while the alto is only used by the viola and a few other instruments.

Individual clefs

Here follows a complete list of the clefs, along with a list of instruments and voice parts notated with them. Each clef is shown in its proper position on the stave, followed by its reference note.
An obelisk (†) after the name of a clef indicates that the clef is no longer in common use.

G-clefs

Treble clef

Diatonic scale on C, treble clef.  Play (help·info)

Where the G-clef is placed on the second line of the stave, it is called the treble clef. This is the most common clef used today, the first clef that those studying music generally learn,^{[citation needed]} and the only G-clef still in use. For this reason, the terms G-clef and treble clef are often seen as synonymous. The treble clef was historically used to mark a treble, or pre-pubescent, voice part.
Among the instruments that use treble clef are the violin, flute, oboe, bagpipe, cor anglais, all clarinets, all saxophones, horn, trumpet, cornet, vibraphone, xylophone, mandolin, recorder; it is also used for the guitar, which sounds an octave lower than written, as well as the euphonium and baritone horn, both of which sound a major ninth lower. Treble clef is the upper stave of the grand stave used for harp and keyboard instruments. It is also sometimes used, along with tenor clef, for the highest notes played by bass-clef instruments such as the cello, double bass (which sounds an octave lower), bassoon, and trombone. The viola also sometimes uses treble clef for very high notes. Treble clef is used for the soprano, mezzo-soprano, alto, contralto and tenor voices. The tenor voice sounds an octave lower, and is often written using an octave clef (see below) or double-treble clef.

French violin clef^†

Diatonic scale on C, French violin clef.  Play (help·info)

In the seventeenth and eighteenth centuries, a special clef was used for violin music, particularly that published in France. For this reason it is known as the French clef or French violin clef although it was more commonly used for flute music.^[2] The G-clef is placed on the first line of the stave and is identical to the bass clef transposed up two octaves.

F-clefs

Bass clef

Diatonic scale on C, bass clef.  Play (help·info)

When the F-clef is placed on the fourth line, it is called the bass (/beɪs/ BAYSS) clef. This is the only F-clef used today so that the terms "F-clef" and "bass clef" are often regarded as synonymous.
This clef is used for the cello, euphonium, double bass, bass guitar, bassoon, contrabassoon, trombone, baritone horn, tuba, and timpani. It is also used for the lowest notes of the horn, and for the baritone and bass voices. Tenor voice is notated in bass clef when the tenor and bass are written on the same stave. Bass clef is the bottom clef in the grand stave for harp and keyboard instruments. The contrabassoon, double bass, and electric bass sound an octave lower than the written pitch; no notation is usually made of this fact, but some composers/publishers will place an "8" beneath the clef for these instruments on the conductor's full score to differentiate from instruments that naturally sound within the clef (see "Octave clefs" below).

Baritone clef^†

Diatonic scale on C, baritone F-clef.  Play (help·info)

When the F-clef is placed on the third line, it is called the baritone clef.
This clef was used for the left hand of keyboard music (particularly in France; see Bauyn manuscript) as well as the baritone part in vocal music.
The baritone clef has the less common variant as a C clef placed on the 5th line which is exactly equivalent (see below).

Sub-bass clef^†

Where the F-clef is placed on the fifth line, it is called the sub-bass clef. It is identical to the treble clef transposed down 2 octaves.
This clef was used by Johannes Ockeghem and Heinrich Schütz to write low bass parts, making a late appearance in Bach's Musical Offering.

C-clefs

Alto clef

Diatonic scale on C, alto clef.  Play (help·info)

Where the C-clef is placed on the third line of the stave, it is called the alto or viola clef.
This clef is currently used for the viola, the viola da gamba, the alto trombone, and the mandola. It is also associated with the countertenor voice and therefore called the counter-tenor (or countertenor) clef,^[3] A vestige of this survives in Sergei Prokofiev's use of the clef for the cor anglais, as in his symphonies. It occasionally turns up in keyboard music to the present day (for example, in Brahms's Organ Chorales, John Cage's Dream for piano).

Tenor clef

Diatonic scale on C, tenor clef.  Play (help·info)

Where the C-clef is placed on the fourth line of the stave, it is called the tenor clef.
This clef is used for the upper ranges of the bassoon, cello, euphonium, double bass, and trombone. These instruments use bass clef for their low- to mid-ranges; treble clef is also used for their upper extremes. Where used for the double bass, the sound is an octave lower than the written pitch. The tenor violin parts were also drafted in this clef (see e.g. Giovanni Battista Vitali's Op. 11). Formerly, it was used by the tenor part in vocal music but its use has been largely supplanted^[why?] either with an octave version of the treble clef where written alone or the bass clef where combined on one stave with the bass part.

Baritone clef^†

Diatonic scale on C, baritone C-clef.  Play (help·info)

Where the C-clef is placed on the 5th line of the stave, it is called the baritone clef. It is precisely the equivalent to the other more common form of the baritone clef, an F clef placed on the 3rd line (see above).

Mezzo-soprano clef^†

Diatonic scale on C, mezzo-soprano clef.  Play (help·info)

Where the C-clef is placed on the second line of the stave, it is called the mezzo-soprano clef.

Soprano clef^†

Diatonic scale on C, soprano clef.  Play (help·info)

Where the C-clef is placed on the first line of the stave, it is called the soprano clef.
This clef was used for the right hand of keyboard music (particularly in France; see Bauyn manuscript) as well as in vocal music for sopranos, and sometimes in high viola da gamba^{[clarification needed]} parts alongside the alto clef.^{[citation needed]}

Other clefs

Octave clefs

Three types of suboctave treble clef showing middle C

Diatonic scale on C, suboctave clef.  Play (help·info)

Diatonic scale on C, "sopranino" clef.  Play (help·info) (this is one octave higher than the treble clef without an 8)

Starting in the 18th-century treble clef has been used for transposing instruments that sound an octave lower, such as the guitar; it has also been used for the tenor voice. To avoid ambiguity, modified clefs are sometimes used, especially in the context of choral writing; of those shown, the C clef on the third space, easily confused with the tenor clef, is the rarest.
This is most often found in tenor parts in SATB settings, in which a treble clef is written with the numeral 8 below it, indicating that the pitches sound an octave below the written value. As the true tenor clef has fallen into disuse in vocal writings, this "octave-dropped" treble clef is often called the tenor clef. The same clef is sometimes used for the octave mandolin. In some scores, the same concept is construed by using a double clef—two G-clefs overlapping one another.
Tenor banjo is commonly notated in treble clef. However, notation varies between the written pitch sounding an octave lower (as in guitar music and called octave pitch in most tenor banjo methods) and music sounding at the written pitch (called actual pitch). An attempt has been made to use a treble clef with a diagonal line through the upper half of the clef to indicate octave pitch, but this is not always used.
At the other end of the spectrum, treble clefs with an 8 positioned above the clef may be used for the piccolo, penny whistle, soprano and sopranino recorder, and other high woodwind parts. A treble clef with a 15 above (sounding two octaves above the standard treble clef) is used for the garklein (sopranissimo) recorder, whose lowest note is two octaves above middle C.
The F clef can also be notated with an octave marker. The F clef notated to sound an octave lower is used for contrabass instruments such as the double bass and contrabassoon. The F clef notated to sound an octave higher is used for the bass recorder. However, both of these are extremely rare (and, in fact, the countertenor clef is largely intended to be humorous as with the works of P.D.Q. Bach). In Italian scores up to Gioachino Rossini's Overture to William Tell, the cor anglais was written in bass clef an octave lower than sounding.^[4] The unmodified bass clef is so common that performers of instruments and voice parts whose ranges lie below the stave simply learn the number of ledger lines for each note through common use, and if a line's true notes lie significantly above the bass clef the composer or publisher will often simply write the part in either the treble clef or notated an octave down under an 8va bracket.
Use of octave-marked clefs appears to have increased as computers have become more important in musical transcription. Performers will normally know the right octave to use with or without the octave marking. However, the appropriate use of octave marking in score editing software ensures that music files (such as MIDI files) generate tones in their proper octaves.

Neutral clef

Simple quadruple drum pattern on a rock drum kit.  Play (help·info)

The neutral or percussion clef is not a clef in the same sense that the F, C, and G clefs are. It is simply a convention that indicates that the lines and spaces of the stave are each assigned to a percussion instrument with no precise pitch. With the exception of some common drum-kit and marching percussion layouts, the keying of lines and spaces to instruments is not standardised, so a legend or indications above the stave are necessary to indicate what is to be played. Percussion instruments with identifiable pitches do not use the neutral clef, and timpani (notated in bass clef) and mallet percussion (noted in treble clef or on a grand stave) are usually notated on different staves than unpitched percussion.
Staves with a neutral clef do not always have five lines. Commonly, percussion staves only have one line, although other configurations can be used.
The neutral clef is sometimes used where non-percussion instruments play non-pitched extended techniques, such as hitting the body of a violin, violoncello or acoustic guitar, or where a vocal choir is instructed to clap, stomp, or snap, but more often the rhythms are written with X marks in the instrument's normal stave with a comment placed above as to the appropriate rhythmic action.

Tablature

Diatonic scale on C, guitar tablature and stave notation (suboctave is assumed).  Play (help·info)

For guitars and other fretted instruments, it is possible to notate tablature in place of ordinary notes. In this case, a TAB-sign is often written instead of a clef. The number of lines of the stave is not necessarily five: one line is used for each string of the instrument (so, for standard six-stringed guitars, six lines would be used, four lines for the traditional bass guitar). Numbers on the lines show on which fret the string should be played. This Tab-sign, like the Percussion clef, is not a clef in the true sense, but rather a symbol employed instead of a clef.

History

Originally, instead of a special clef symbol, the reference line of the stave was simply labeled with the name of the note it was intended to bear: F and C and, more rarely, G. These were the most often-used 'clefs', or litterae clavis (key-letters), in Gregorian chant notation. Over time the shapes of these letters became stylised, leading to their current versions.
Many other clefs were used, particularly in the early period of chant notation, including most of the notes from the low Γ (gamma, the note written today on the bottom line of the bass clef) up to the G above middle C, written with a small letter g, and including two forms of lowercase b (for the note just below middle C): round for B♭, and square for B♮. In order of frequency of use, these clefs were: F, c, f, C, D, a, g, e, Γ, B, and the round and square b.^[5]

Early forms of the G clef—the third combines the G and D clefs vertically

In the polyphonic period up to 1600, unusual clefs were occasionally used for parts with extremely high or low written tessituras. For very low bass parts, the Γ clef is found on the middle, fourth, or fifth lines of the stave (e.g., in Pierre de La Rue’s Requiem and in a mid-16th-century dance book published by the Hessen brothers); for very high parts, the high-D clef (d), and the even higher ff clef (e.g., in the Mulliner Book) were used to represent the notes written on the fourth and top lines of the treble clef, respectively.^[6]
Varying shapes of different clefs persisted until very recent times. The F-clef was, until as late as the 1980s in some cases (such as hymnals), or in British and French publications, written like this:
In printed music from the 16th and 17th centuries, the C clef often assumed a ladder-like form, in which the two horizontal rungs surround the stave line indicated as C: ; this form survived in some printed editions (see this example, written in four-part men's harmony and positioned to make it equivalent to an octave G clef) into the 20th century.
The C-clef was formerly written in a more angular way, sometimes still used, or an, even more, simplified K-shape, when writing the clef by hand:
In modern Gregorian chant notation, the C clef is written (on a four-line stave) in the form and the F clef as
The flourish at the top of the G-clef probably derives from a cursive S for "sol", the name for "G" in solfege.^[7]

Vocal music can be contracted into two staves, using the treble and bass clefs.

C clefs (along with G, F, Γ, D, and A clefs) were formerly used to notate vocal music. Nominally, the soprano voice parts were written in first- or second-line C clef (soprano clef or mezzo-soprano clef) or second-line G clef (treble clef), the alto or tenor voices in third-line C clef (alto clef), the tenor voice in fourth-line C clef (tenor clef) and the bass voice in third-, fourth- or fifth-line F clef (baritone, bass, or sub-bass clef).
Despite those small variations, until the 19th century, the most common arrangement for vocal music used the following clefs:^{[contradictory]}

• Soprano = soprano clef (first-line C clef)
• Alto = alto clef (third-line C clef)
• Tenor = tenor clef (fourth-line C clef)
• Bass = bass clef (fourth-line F clef)

In more modern publications, four-part harmony on parallel staves is usually written more simply as:

• Soprano = treble clef (second-line G clef)
• Alto = treble clef
• Tenor = treble clef with an 8 below or a double treble clef. Many pieces, particularly those from before the 21st century, use an unaltered treble clef, with the expectation the tenors will still sing an octave lower than notated.
• Bass = bass clef (fourth-line F clef)

This may be reduced to two staves, the soprano/alto stave with a treble clef, and tenor/bass stave marked with the bass clef.

Further uses

Clef combinations played a role in the modal system toward the end of the 16th century, and it has been suggested certain clef combinations in the polyphonic music of 16th-century vocal polyphony are reserved for authentic (odd-numbered) modes, and others for plagal (even-numbered) modes,^[8][9] but the precise implications have been the subject of much scholarly debate.^{[10][11][12][13]}
Music can be transposed at sight if a different clef is mentally substituted for the written one. For example, to play an A-clarinet part, a B♭-clarinet player may mentally substitute tenor clef for the written treble clef. Concert-pitch music in bass clef can be read on an E♭ instrument as if it were in treble clef. (Notes will not always sound in the correct octave.) The written key signature must always be adjusted to the proper key for the instrument being played.

See also

• Chiavette
• Clef transposition

Adaptive group bridge estimation for high-dimensional partially linear ...

https://link.springer.com/article/10.1186/s13660-017-1432-x

by X Wang - ‎2017 - ‎Cited by 1 - ‎Related articles

Y=\mathbf {x}^{T}\boldsymbol {\beta }+f(U)+\varepsilon, $$ .... with \(\mathbf {g}(U_{i})=E(\mathbf {x}_{i}|U_{i})\) and \(\widetilde {\mathbb {X}}=(\tilde ... Let \(\mathcal {A}= \{ 1\leq j \leq p_{n}: \|\boldsymbol {\beta }_{0j}\| \neq0\}\) be the index set of the .... {\beta }_{0} \Vert =O_{P}\bigl( \sqrt{n^{-1}p_{n}}+\sqrt{p_{n}}q_{n}^{-r} \bigr).

Stabilized finite volume element method for the 2D nonlinear ...

https://link.springer.com/article/10.1186/s13661-017-0862-1

by H Xia - ‎2017 - ‎Cited by 1 - ‎Related articles

Seek \(\boldsymbol {\sigma}=(\sigma _{l,n})_{2\times2}\) and \(\boldsymbol ... Recently, a stabilized mixed finite element (SMFE) method of Problem I has been posed in [7]. ... {u})=0, \quad \forall\vartheta\in \boldsymbol {\mathcal {M}}, \\ \boldsymbol .... {u}(t_{j})-\boldsymbol {u}^{j}\bigr) \bigr\Vert _{0}^{2}+ \bigl\Vert p(t_{j})-p^{j} ...

Unsteady wave pattern generation by water striders

https://www.cambridge.org/core/product/.../core-reader

3 days ago - Panels (a,d,g,j,m) represent the experimental surface elevation generated ... (3.13) (Bühler 2007); (c,f,i,l,o) show the results for continuous forcing in shallow ..... pressure field $p_{ext}(x,y)$ , with $\hat{\unicode[STIX]{x1D701}}(k)$ and ..... The velocity potential $\unicode[STIX]{x1D719}(\boldsymbol{r},t)$ is ...

Spatial structure of shock formation - Cambridge University Press

https://www.cambridge.org/core/product/.../core-reader

May 5, 2017 - (1.1) $$\begin{eqnarray}\displaystyle u_{t}+uu_{x}=0, & & \displaystyle\end{eqnarray}$$ ... we must have $t_{c}(y^{\prime })-t_{0}=ay^{\prime 2}+O(y^{3})$ ; the .... $\unicode[STIX]{x1D70C}$ and pressure $p$ to be described by the ...... the mass flux $\boldsymbol{j}=\unicode[STIX]{x1D70C}\boldsymbol{v}$ ...

Asymptotic analysis of non-autonomous discrete hungry integrable ...

https://academic.oup.com/integrablesystems/article/3/1/xyy001/4827627

by M Shinjo - ‎2018

Jan 27, 2018 - Asymptotic analysis of non-autonomous discrete hungry integrable systems ..... \begin{align} f_{s}^{(t)}=\sum_{p=1}^{m}c_{p}^{(s\ \text{mod}\ ..... \displaystyle \sum_{p=1}^m\tilde{c}_p^{(j)}\rho_p^{(t)}\lambda_p^\ell &\displaystyle .... and $$\boldsymbol{z}_k:=(1,z,\dots,z^{k-1})^{\top}$$, we can express the ...

New developments for modern celestial mechanics – I. General ...

https://academic.oup.com/mnras/article/435/3/2187/1024281

by RA Mardling - ‎2013 - ‎Cited by 19 - ‎Related articles

where $${\boldsymbol r}$$ is the position of body 2 relative to body 1, .... \begin{equation} X_{n^{\prime }}^{-(l+1),m}(e_{\rm o})=\frac{1}{2\pi }\int _0^{2\pi }\frac{{\rm .... [T_{20}+T_{40}+\ldots ] +[T_{31}+T_{51}+\ldots ] +[T_{22}+T_{42}+\ldots .... where Pi and Po are the inner and outer orbital periods, Tp ≡ (1 − eo)^3/2Po is the ...

Searches related to {\displaystyle {\boldsymbol {T}}_{o}(p_{i,j})=p_{i,j}+t_{o}}

angular velocity formula

transposition music

angular velocity definition

types of transposition in music

langevin equation derivation

transposing music chart

overdamped langevin equation

angular velocity example

 William Sealy Gosset, who developed the "t-statistic"

t-statistic

From Wikipedia, the free encyclopedia

Jump to navigation Jump to search

This article does not cite any sources. Please help improve this article by adding citations to reliable sources. Unsourced material may be challenged and removed. (February 2011) (Learn how and when to remove this template message)

In statistics, the t-statistic is the ratio of the departure of the estimated value of a parameter from its hypothesized value to its standard error. It is used in hypothesis testing. For example, it is used in estimating the population mean from a sampling distribution of sample means if the population standard deviation is unknown.

Contents

• 1 Definition and features
• 2 Use
  • 2.1 Prediction
• 3 History
• 4 Related concepts
• 5 See also
• 6 References
• 7 External links

Definition and features

Let ${\displaystyle \scriptstyle {\widehat {\beta }}}$ be an estimator of parameter β in some statistical model. Then a t-statistic for this parameter is any quantity of the form

${\displaystyle t_{\widehat {\beta }}={\frac {{\widehat {\beta }}-\beta _{0}}{\operatorname {s.e.} ({\widehat {\beta }})}}}$

where β₀ is a non-random, known constant which may or may not match the actual unknown parameter value β, and ${\displaystyle \operatorname {s.e.} ({\widehat {\beta }})}$ is the standard error of the estimator ${\displaystyle \scriptstyle {\widehat {\beta }}}$ for β.
By default, statistical packages report t-statistic with β₀ = 0 (these t-statistics are used to test the significance of corresponding regressor). However, when t-statistic is needed to test the hypothesis of the form H₀: β = β₀, then a non-zero β₀ may be used.
If ${\displaystyle \scriptstyle {\widehat {\beta }}}$ is an ordinary least squares estimator in the classical linear regression model (that is, with normally distributed and homoscedastic error terms), and if the true value of the parameter β is equal to β₀, then the sampling distribution of the t-statistic is the Student's t-distribution with (n − k) degrees of freedom, where n is the number of observations, and k is the number of regressors (including the intercept).
In the majority of models the estimator ${\displaystyle \scriptstyle {\widehat {\beta }}}$ is consistent for β and is distributed asymptotically normally. If the true value of the parameter β is equal to β₀ and the quantity ${\displaystyle \scriptstyle \operatorname {s.e.} ({\widehat {\beta }})}$ correctly estimates the asymptotic variance of this estimator, then the t-statistic will asymptotically have the standard normal distribution.
In some models the distribution of the t-statistic is different from the normal distribution, even asymptotically. For example, when a time series with a unit root is regressed in the augmented Dickey–Fuller test, the test t-statistic will asymptotically have one of the Dickey–Fuller distributions (depending on the test setting).

Use

Main article: t-test

Most frequently, t statistics are used in Student's t-tests, a form of statistical hypothesis testing, and in the computation of certain confidence intervals.
The key property of the t statistic is that it is a pivotal quantity – while defined in terms of the sample mean, its sampling distribution does not depend on the population parameters, and thus it can be used regardless of what these may be.
One can also divide a residual by the sample standard deviation:

${\displaystyle g(x,X)={\frac {x-{\overline {X}}}{s}}}$

to compute an estimate for the number of standard deviations a given sample is from the mean, as a sample version of a z-score, the z-score requiring the population parameters.

Prediction

Further information: Prediction interval § Unknown mean, unknown variance

Given a normal distribution ${\displaystyle N(\mu ,\sigma ^{2})}$ with unknown mean and variance, the t-statistic of a future observation ${\displaystyle X_{n+1},}$ after one has made n observations, is an ancillary statistic – a pivotal quantity (does not depend on the values of μ and σ²) that is a statistic (computed from observations). This allows one to compute a frequentist prediction interval (a predictive confidence interval), via the following t-distribution:

${\displaystyle {\frac {X_{n+1}-{\overline {X}}_{n}}{s_{n}{\sqrt {1+n^{-1}}}}}\sim T^{n-1}}$

Solving for ${\displaystyle X_{n+1}}$ yields the prediction distribution

${\displaystyle {\overline {X}}_{n}+s_{n}{\sqrt {1+n^{-1}}}\cdot T^{n-1}}$

from which one may compute predictive confidence intervals – given a probability p, one may compute intervals such that 100p% of the time, the next observation ${\displaystyle X_{n+1}}$ will fall in that interval.

History

Further information: Student's t-test

The term "t-statistic" is abbreviated from "hypothesis test statistic",^{[citation needed]} while "Student" was the pen name of William Sealy Gosset, who introduced the t-statistic and t-test in 1908, while working for the Guinness brewery in Dublin, Ireland.

Related concepts

• z-score (standardization): If the population parameters are known, then rather than computing the t-statistic, one can compute the z-score; analogously, rather than using a t-test, one uses a z-test. This is rare outside of standardized testing.
• Studentized residual: In regression analysis, the standard errors of the estimators at different data points vary (compare the middle versus endpoints of a simple linear regression), and thus one must divide the different residuals by different estimates for the error, yielding what are called studentized residuals.